Vectors and Linear Transformations
Quiz
Q1
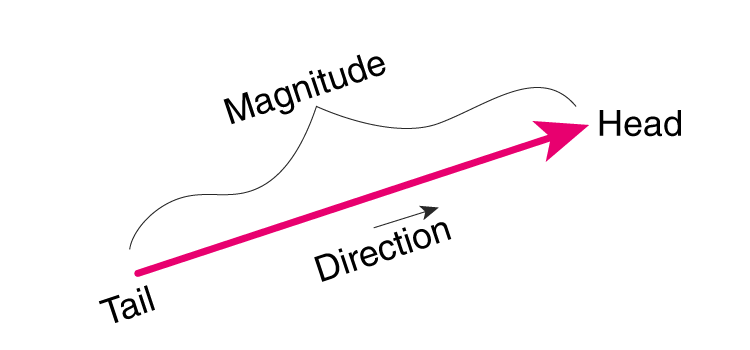
Which of the following options is true for a vector?
Choice
- A vector has a shape and weight.
- A vector has only a magnitude.
- A vector has only direction.
- A vector has a magnitude and direction.
Answer
4
Q2
Compute the sum of the vectors $\vec u$ and $\vec v$.

Hint: The sum vector is the diagonal in a parallelogram formed by the two vectors, $\vec u = (1,3)$ and $\vec v = (6, 2)$
Choice
- $\vec u + \vec v = (7,5)$
- $\vec u + \vec v = 20$
- $\vec u + \vec v = 3$
- $\vec u + \vec v = (6,3)$
Answer
1
Correct! $\vec u + \vec v = ((1 + 6), (3+2))$
Q3
Using the image from question 2, compute the difference of the vectors $\vec u$ and $\vec v$.
Choice
- $\vec u - \vec v = (5,1)$
- $\vec u - \vec v = (-1,5)$
- $\vec u - \vec v = 3$
- $\vec u - \vec v = (-5,1)$
Answer
4
Correct! $\vec u - \vec v = ((1 - 6), (3-2))$
Q4
Calculate the dot product of the given vectors $\vec a \cdot \vec b$ and select the correct answer.
$$ \vec a = \left[ \begin{array}{c}-1 \\ 5 \\2 \end{array} \right], \,\,\vec b = \left[ \begin{array}{c}-3\\6\\-4 \end{array} \right] $$
Choice
- $\left[ \begin{array}{c}-3 \\ 30 \\-8 \end{array} \right]$
- 30
- 25
- $\left[ \begin{array}{c}1\\0\\1 \end{array} \right]$
Answer
3
Correct! By applying the formula you saw in the video "The dot product" as follows:
$\vec a \cdot \vec b = a_1 \cdot b_1 + a_2 \cdot b_2 + a_3 \cdot b_3$, you have:
$\vec a \cdot \vec b = (-1)\cdot(-3) + 5\cdot 6 + 2\cdot (-4) = 3 + 30 - 8 = 25$
Q5
Which of the following is true, if $\vec a \cdot \vec a = 0$ and $\vec a \cdot \vec b = 0$?
Choice
- $\vec a = 0, \vec b = 0$
- $\vec a \cdot \vec a = 1$
- $\vec a = 0, \vec b=$ any vector
- $\vec a \ne 0, \vec b =0$
Answer
3
Correct! Since $\vec a$ is set to 0 by definition in the problem, then $\vec a$ cannot be any vector, but 0, leaving the vector $\vec b$ to be any vector. Even if $\vec b$ is a vector different than 0, the dot product of $\vec a$ and $\vec b$ is 0, fulfilling both conditions in the problem.
Q6
Which of the following is the correct representative system of equation for the given dot product:

The image above represents the following:
$$ \left[ \begin{array}{ccc}3&5&1 \end{array}\right] \cdot \left[ \begin{array}{c}x\\y\\z \end{array}\right] = 10 \\ \left[ \begin{array}{ccc}7&-2&4 \end{array}\right] \cdot \left[ \begin{array}{c}x\\y\\z \end{array}\right] = 2 \\ \left[ \begin{array}{ccc}-6&3&2 \end{array}\right] \cdot \left[ \begin{array}{c}x\\y\\z \end{array}\right] = 15 $$
Choice
1
$$ \left\{\begin{aligned}3x + 5y + z & =2 \\7x -2y + 4z & =1 \\-6x + 3y + 2z&=20\end{aligned}\right. $$
2
$$ \left\{\begin{aligned}3x + 5y + z & =10 \\7x -2y + 4z & =2 \\-6x + 3y + 2z&=5\end{aligned}\right. $$
3
$$ \left\{\begin{aligned}3x + -2y + 4z & =10 \\7x -2y + 4z & =2 \\-6x + 3y + 2z&=5\end{aligned}\right. $$
Answer
2
Correct!
The first element (3) gets multiplied with the first element of the vector (x), the second element (5) gets multiplied with the second element of the vector (y), and the third element (1) gets multiplied with the third element of the vector (z). This will form the first equation which is equal to 10 in the system of equations. Apply the same for the remaining elements to form the two other equations.
All the information here is based on the Linear Algebra for Machine Learning and Data Science | Coursera from DeepLearning.AI
'Coursera > Mathematics for ML and Data Science' 카테고리의 다른 글
| Linear Algebra for Machine Learning and Data Science (12) (1) | 2024.05.17 |
|---|---|
| Linear Algebra for Machine Learning and Data Science (11) (0) | 2024.05.16 |
| Linear Algebra for Machine Learning and Data Science (9) (1) | 2024.05.05 |
| Linear Algebra for Machine Learning and Data Science (8) (0) | 2024.05.04 |
| Linear Algebra for Machine Learning and Data Science (7) (0) | 2024.05.03 |




 }
}